It have became to be clear that the most minimal unit of the matrix to be concerned with appearances of pentagram without excess and deficiency
was managed on more advanced joint-system, from the newest exploration for specific major relics. (explanation for the most minimal unit)
There is need to grasp the method of 3step-reducing-join as most important fundamental practice.
Almost major relics checked already seem to take in 3step process. However, actually this reducing-join is able to repeat many times.
The newest check of this investigation has confirmed the status of 4step-reducing-join on Tibetan Hevajra-Mandala in 13-century.
This method to join minimal units is effected from natural tonality of adaptation to geometry, because which have no external factor to decide behavior.
The process is just constraining to ownself from the actual rate surfaced on the own figure.
The next figure is illustrating the status finished the 3step-reducing-join of minimal units.
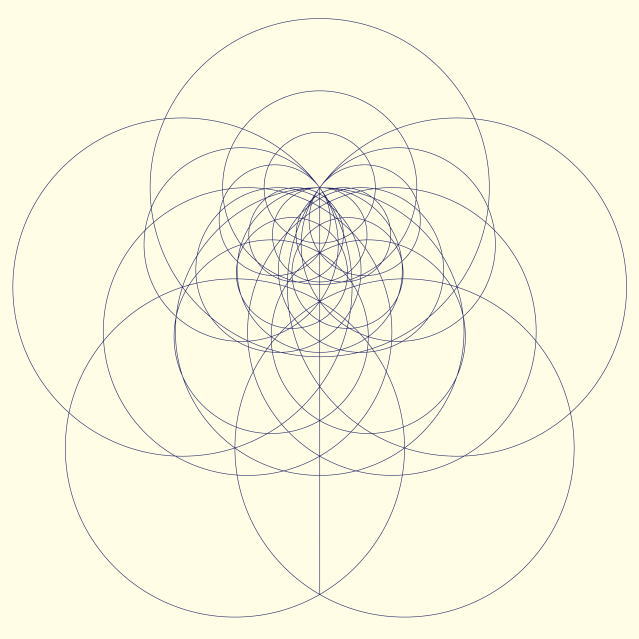
Which status results from the process to overlap minimal units reduced two times in series on a unit as first base by the certain rate.
That schema is next figure.

The concept conducting to the specific rate of reduction is very simple. Which comes into existence only concerning geometric integrity.
It is only necessary the grasp of positions of 2 intersecting points on the axis, in the figure of a minimal unit below.
That 2 points are marking as A and B in there.
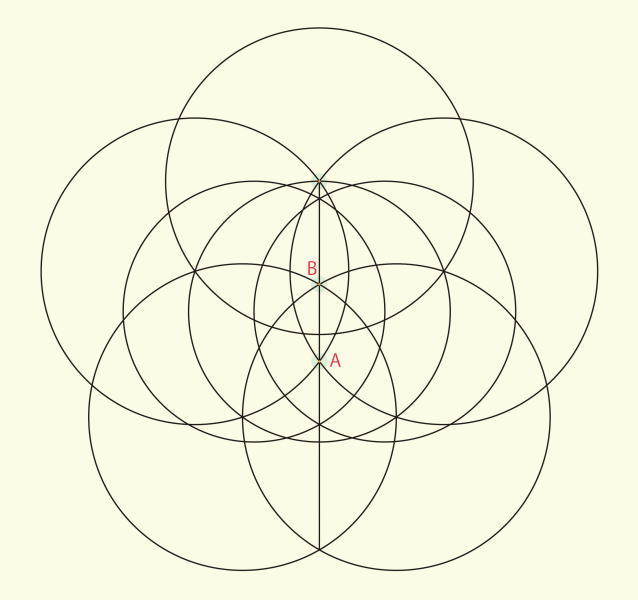
When this reducing overlap process is performed, It needs to gather vertexes being most upper position of each pentagram in units,
in other words, the each center point of most upper circles of minimal units.
In parallel, if A-point of the reduced unit for overlap becomes the same of B-point on the non-reduced unit,
one time practice of this reducing-join has be completed. And this process is able to repeat as a next reducing step.
